Two circles touch externally at a point P. From a point T on the tangent at P, tangents TQ and TR are drawn to the circles with points of contact Q and R respectively. Prove that TQ = TR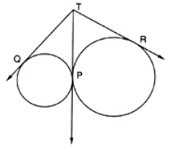
Matching exercise
Match the items on the right to the items on the left.
STEP 1
STEP 2
STEP 3
As TQ and TP are tangents to circle a,And TP and TR are tangents to circle b.By theorem which states that the lengths of the two tangents drawn from external point to a circle are equal.
TQ=TP ...(1)
TP=TR ...(2) From 1 and 2, TQ=TR Hence proved

