Introduction to Trigonometry

Introduction

From very ancient times surveyors, navigators and astronomers have made use of triangles to determine distances that could not be measured directly. This gave birth to the branch of mathematics what we call today as “Trigonometry”.

Hipparchus of Rhodes around 200 BC(BCE), constructed a table of chord lengths for a circle of circumference 360 × 60 = 21600 units which corresponds to one unit of circumference for each minute of arc. For this achievement, Hipparchus is considered as “The Father of Trigonometry” since it became the basis for further development.

Trigonometric Ratios

Let 0° < θ < 90°

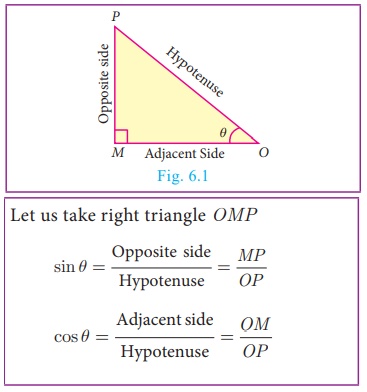
From the above two ratios we can obtain other four trigonometric ratios as follows.

Note

All right triangles with θ as one of the angle are similar. Hence the trigonometric ratios defined through such right angle triangles do not depend on the triangle chosen.

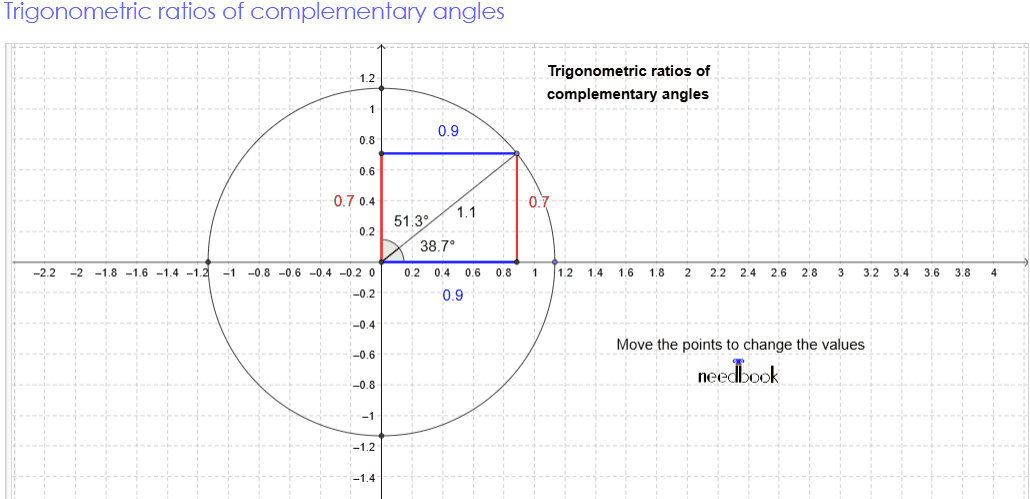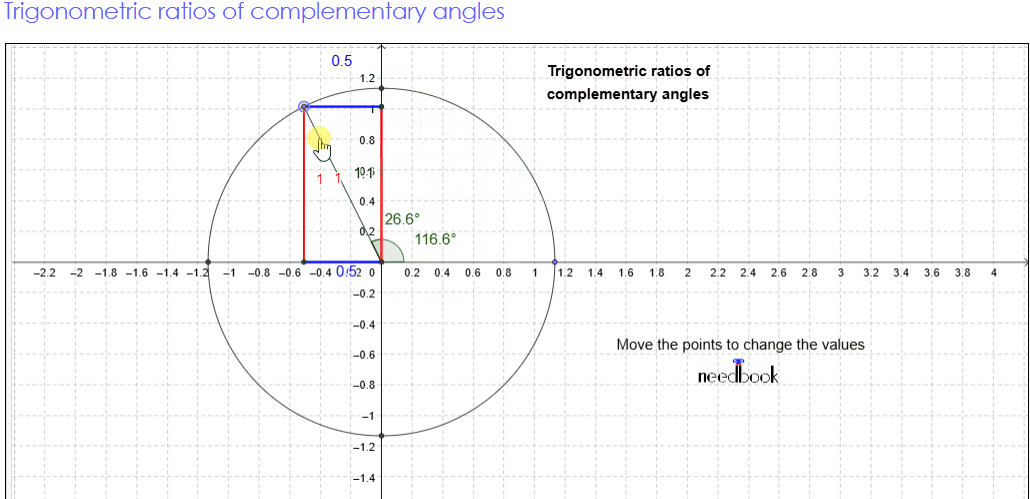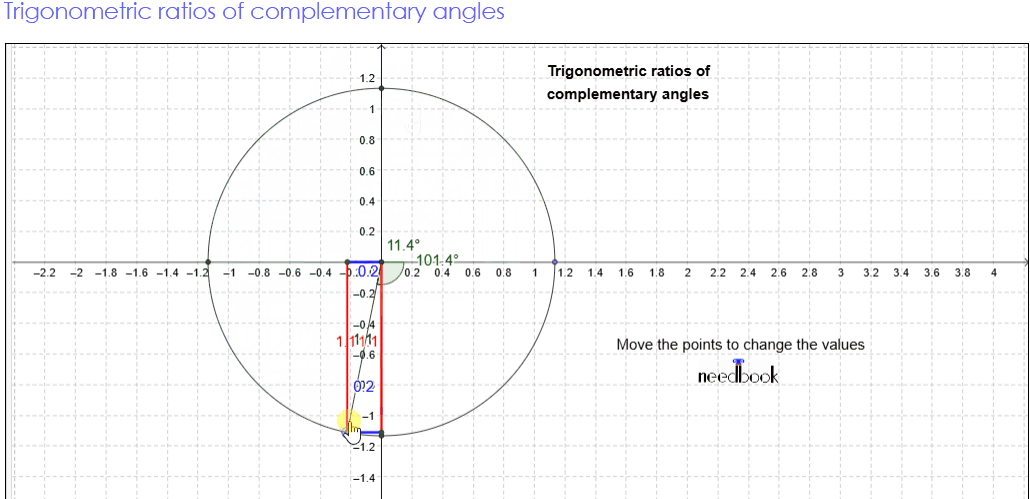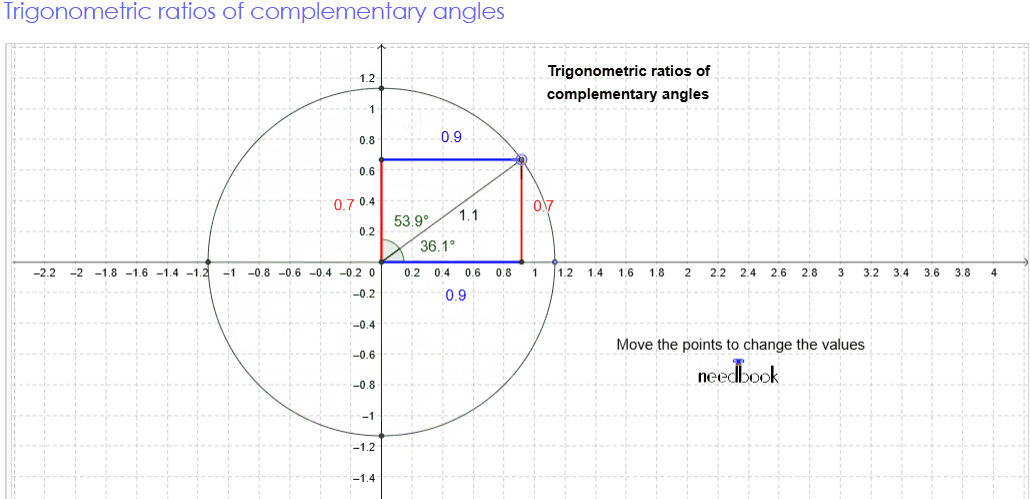
Complementary angle

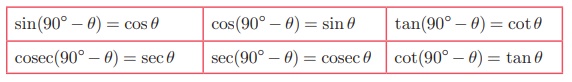
Visual proof of Trigonometric complementary angle

Consider a semicircle of radius 1 as shown in the figure.

Let ∠QOP = θ.

Then ∠QOR =90° − θ, so that OPQR forms a rectangle.

From triangle OPQ , OP/OQ = cos θ

But OQ = radius = 1

Therefore OP = OQ cos θ = cos θ

Similarly, PQ/OQ = sin θ

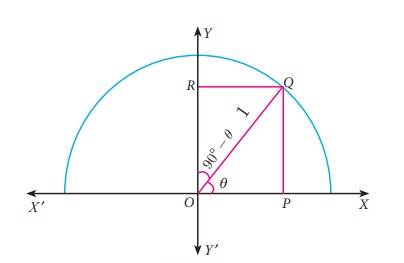
Gives, PQ = OQ sin θ = sin θ (since OQ = 1)

OP = cosθ, PQ = sinθ  … (1)

Now, from triangle QOR,

we have OR/OQ = cos(90° − θ)

Therefore, OR = OQ cos(90° − θ)

So, OR = cos(90° − θ)

Similarly, RQ/OQ = sin(90° − θ)

Then, RQ = sin(90° − θ)

OR= cos(90° − θ) , RQ = sin(90° − θ) … (2)

Since OPQR is a rectangle,

OP = RQ and OR = PQ

Therefore from (1) and (2) we get,

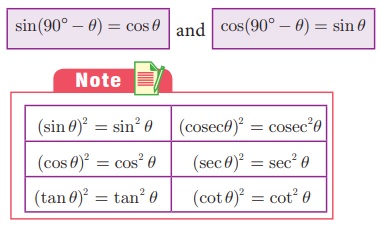
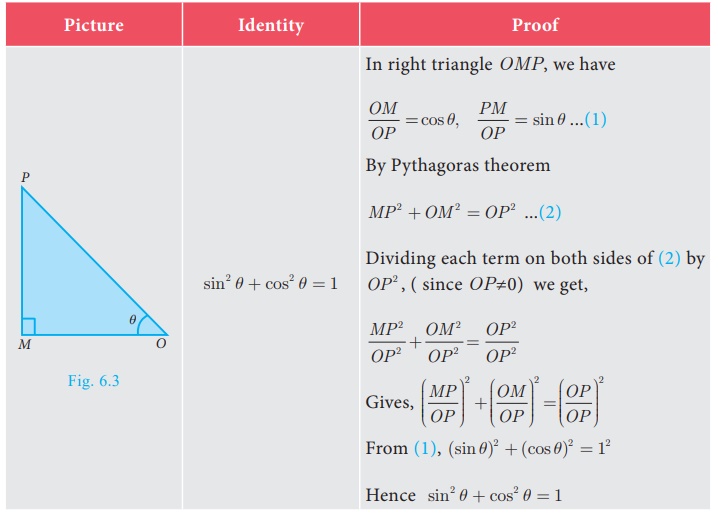
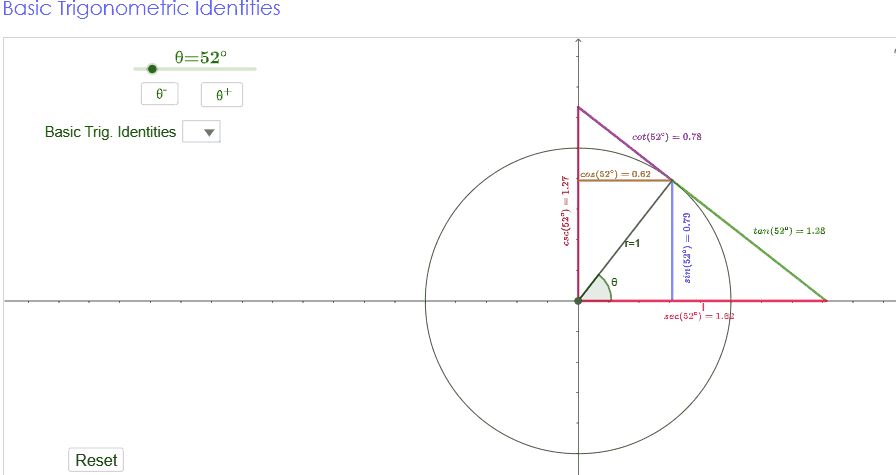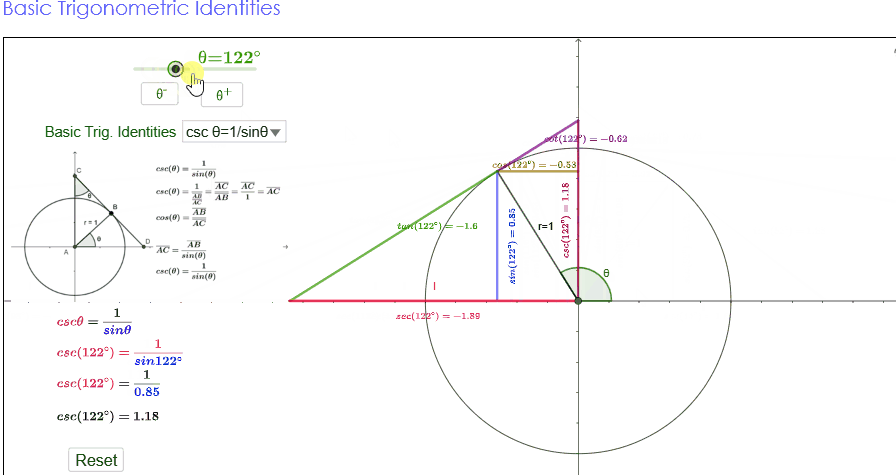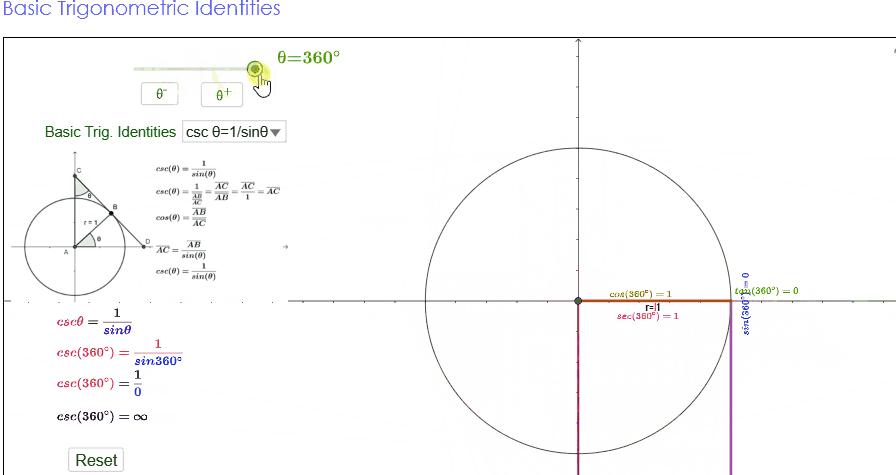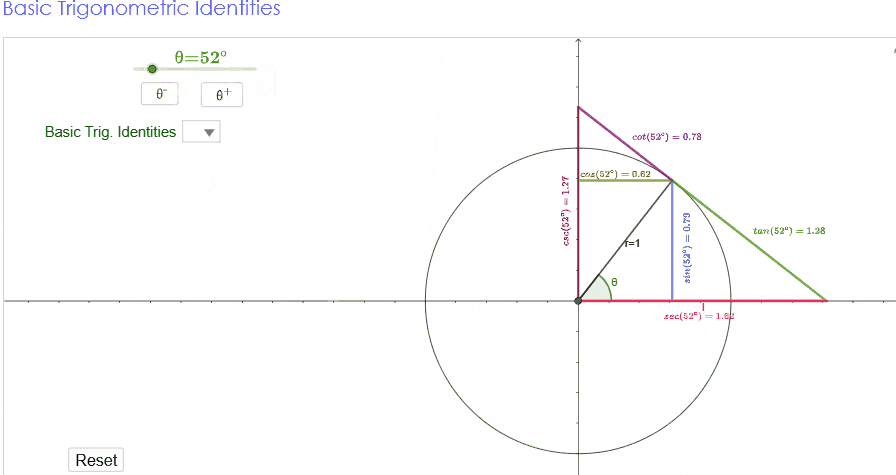
Trigonometric identities

For all real values of θ , we have the following three identities.

(i) sin ² θ + cos² θ=1

(ii) 1 + tan² θ = sec² θ

(iii) 1 + cot² θ = cosec² θ

These identities are termed as three fundamental identities of trigonometry. We will now prove them as follows.

Example 6.1

Prove that tan ² θ − sin² θ = tan ² θ sin² θ

Solution

tan ² θ - sin² θ = tan² θ − 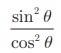. cos² θ

 = tan ² θ(1 − cos ² θ) = tan ² θ sin² θ

Example 6.2

Prove that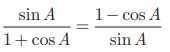 

Solution

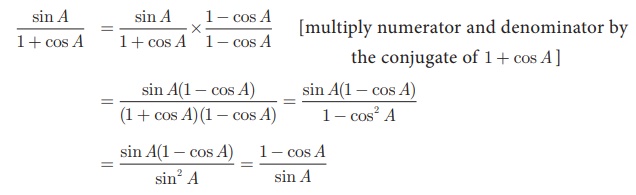
Example 6.3

Prove that 1 + 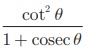 = cosec θ

Solution

Example 6.5 Prove that  = cosec θ + cot θ

Solution

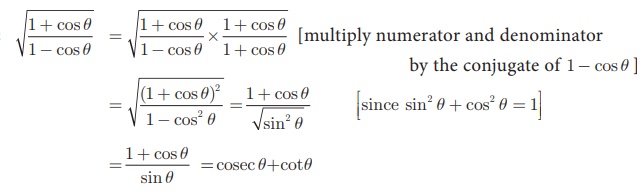
Example 6.6

Prove that 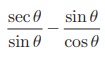 = cot θ

Solution

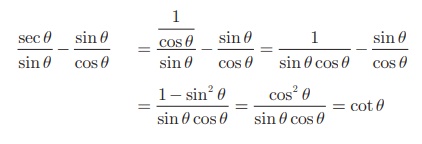
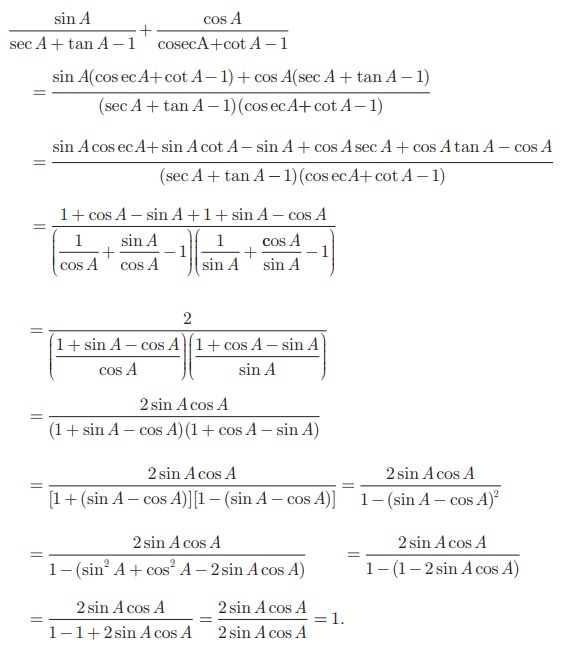
Example 6.14 Prove that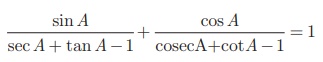 

Solution

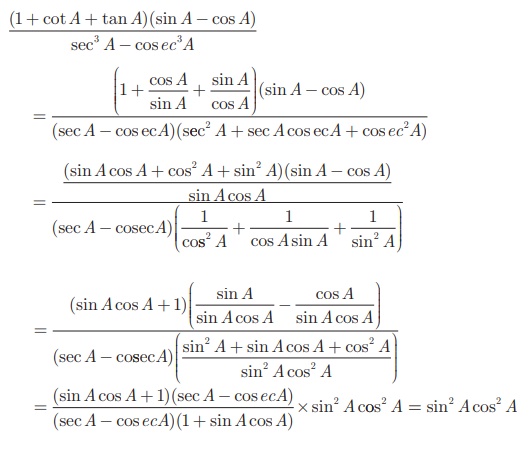
Example 6.16

Prove that 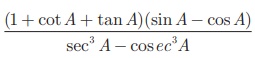 = sin ² A cos² A

Solution

Example 6.17

Solution

Trigonometric Ratios of Some Specific Angles :

For certain specific angles such as 30°, 45° and 60°, which are frequently seen in applications, we can use geometry to determine the trigonometric

Trigonometric Ratios of 30° and 60°

Let ABC be an equilateral triangle whose sides have length a (see the figure given below). Draw AD perpendicular to BC, then D bisects the side BC.

Then,

BD  =  DC  =  a/2

∠BAD  =  ∠DAC  =  30°

Now, in right triangle ADB, ∠BAD  =  30° and BD  =  a/2.

In right triangle ADB, by Pythagorean theorem, 

AB²  =  AD² + BD²

a²  =  AD² + (a/2)²

a² - (a²/4)  =  AD²

3a²/4  =  AD²

√(3a²/4)  =  AD

√3 ⋅ a/2  = m AD

Hence, we can find the trigonometric ratios of angle 30° from the right triangle ADB.

In right triangle ADB, <ABD  =  60°. So, we can determine the trigonometric ratios of angle 60°. 

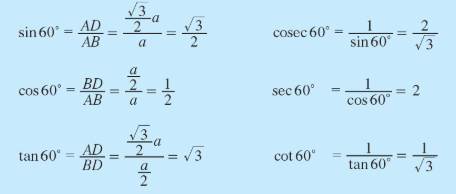
Trigonometric Ratio of 45°

If an acute angle of a right triangle is 45°, then the other acute angle is also 45°. 

Thus the triangle is isosceles. Let us consider the triangle ABC with 

∠B  =  90°

∠A  =  ∠C  =  45°

Then AB  =  BC.

Let AB  =  BC  =  a.

By Pythagorean theorem,

AC²  =  AB² + BC²

AC²  =  a² + a²

AC²  =  2a²

Take square root on each side.

AC  =  a√2

Hence, we can find the trigonometric ratios of angle 45° from the right triangle ABC. 

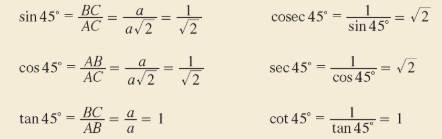
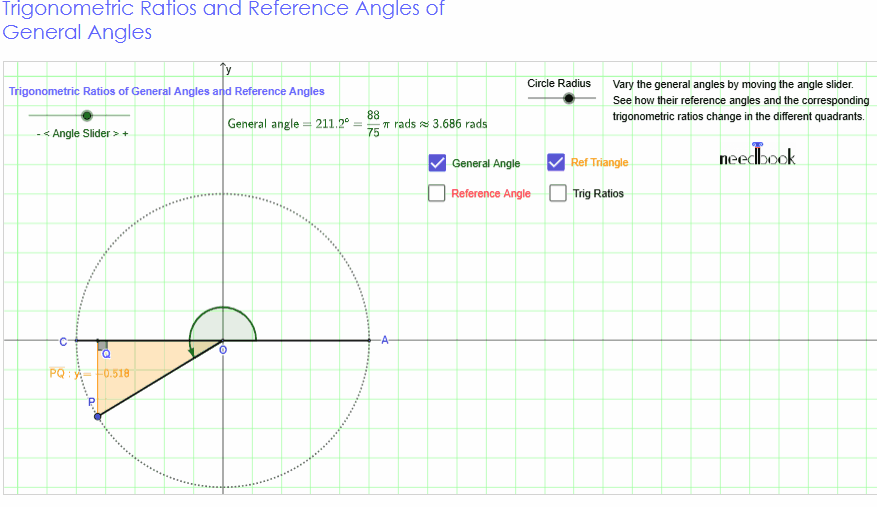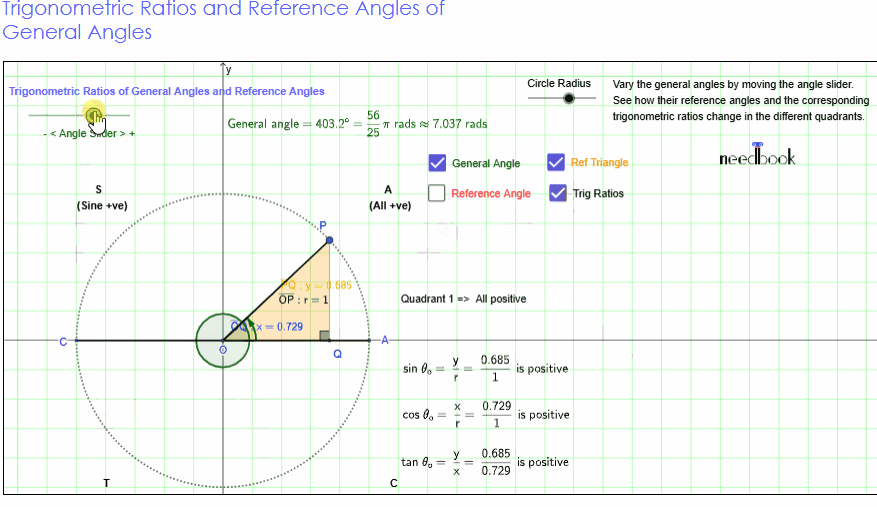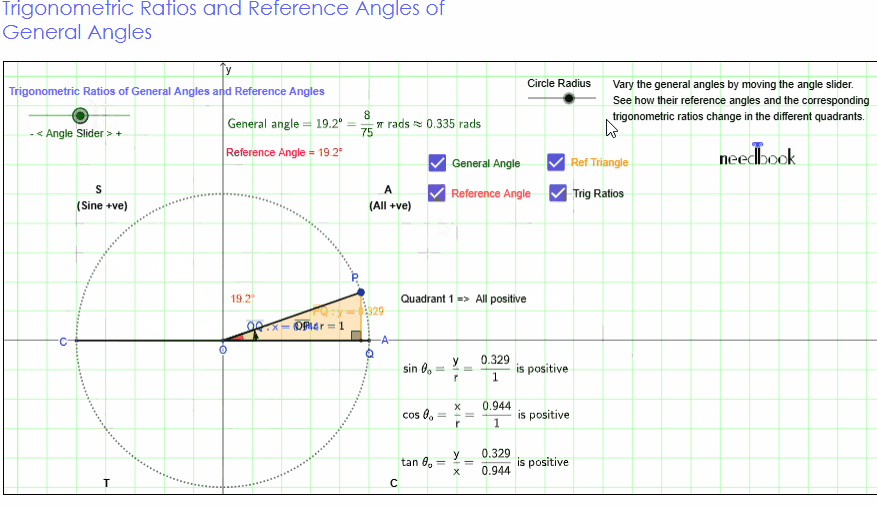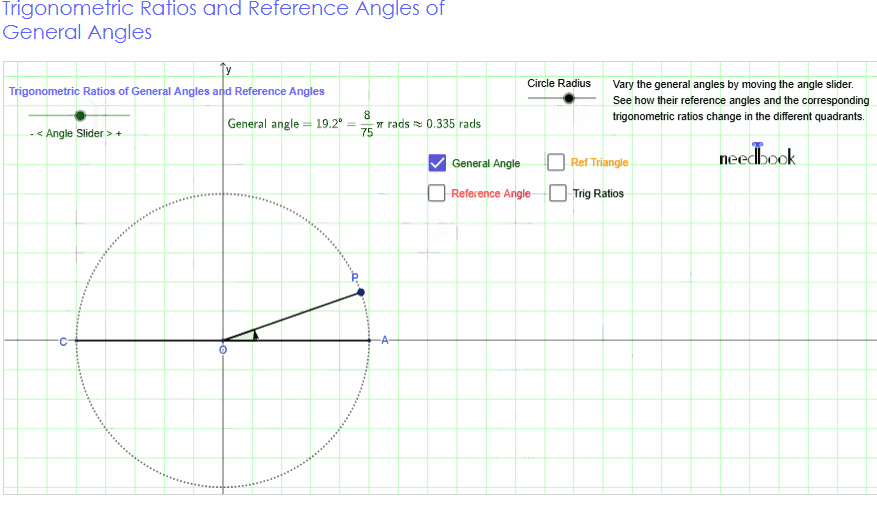
Trigonometric Ratios of 0° and 90°

Consider the figure given below which shows a circle of radius 1 unit centered at the origin.

Let P be a point on the circle in the first quadrant with coordinates (x, y).

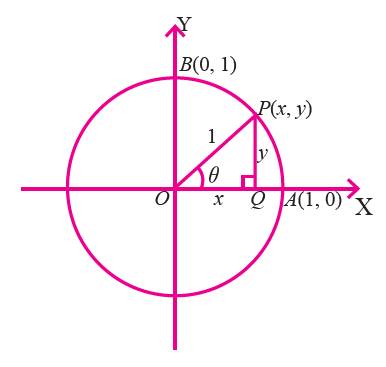
We drop a perpendicular PQ from P to the x-axis in order to form the right triangle OPQ.

Let ∠POQ  =  θ, then 

sin θ  =  PQ / OP  =  y/1  =  y  (y coordinate of P)

cos θ  =  OQ / OP  =  x/1  =  x  (x coordinate of P)

tan θ  =  PQ / OQ  =  y/x

If OP coincides with OA, then angle θ  =  0°.

Since, the coordinates of A are (1, 0), we have

If OP coincides with OB, then angle θ  =  90°.

Since, the coordinates of B are (0, 1), we have

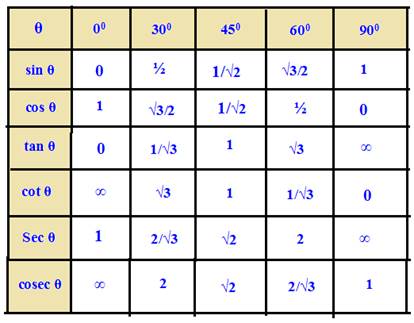
The six trigonometric ratios of angles 0°, 30°, 45°, 60° and 90° are provided in the following table.

Trigonometric Ratios of Complementary Angles

We know that complementary angles are the set of two angles such that their sum is equal to 90°. For example 30° and 60° are complementary to each other as their sum is equal to 90°.

Thus, two angles X and Y are complementary if,

∠X + ∠Y = 90°

In such a condition ∠X is known as the complement of ∠Y and vice-versa.

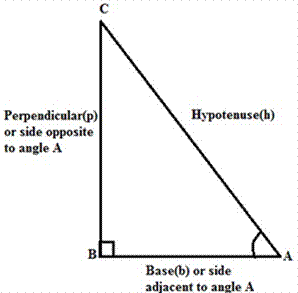
In a right angle triangle, as the measure of the right angle is fixed, the remaining two angles are always complementary as the sum of angles in a triangle is 180°.

The given triangle ∆ABC is right-angled at B; ∠A and ∠C form a complementary pair.

⇒ ∠A + ∠C = 90°

The relationship between the acute angle and the lengths of sides of a right-angle triangle is expressed by trigonometric ratios.

For the given right angle triangle, the  trigonometric ratios of ∠A is given as follows:

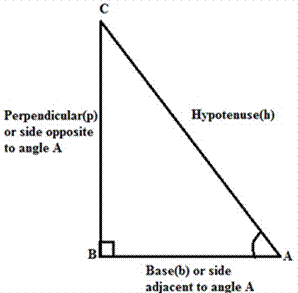
sin A = BCAC

cos A = ABAC

tan A = BCAB

csc A = 1sin A  = ACBC

sec A = 1cos A =  ACAB

cot A = ABBC

The trigonometric ratio of the complement of ∠A i.e., ∠C can be given as:

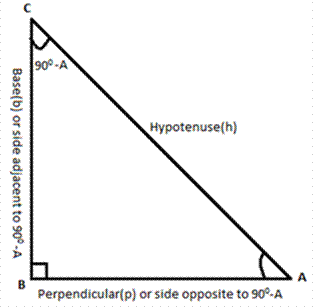
As ∠C = 90°- A (A is used for convenience instead of ∠A ), and the side opposite to 90° – A is AB and the side adjacent to the angle 90°- A is BC as shown in the figure given above.

Therefore,

sin (90°- A) = ABAC

cos (90°- A) = BCAC

tan (90°- A) =  ABBC

csc (90°- A) = 1sin (90° − A) =  ACAB

sec (90°- A) = 1cos (90° − A) =  ACBC

cot (90°- A) =  BCAB

Comparing the above set of ratios with the ratios mentioned earlier, it can be seen that;

sin (90°- A) = cos A ; cos (90°- A) = sin A

tan (90°- A) = cot A; cot (90°- A) = tan A

sec (90°- A) = csc A; csc (90°- A) = sec A

These relations are valid for all the values of a lying between 0° and 90°. It must also be noted that sec 90°,