An airline agrees to charter planes for a group. The group needs at least 160 first class seats and at least 300 tourist class seats. The airlines must use at least two of its model 314 planes which have 20 first class and 30 tourist class seats. The airline will also use some of its model 535 planes which have 20 first class seats and 60 tourist class seats. Each flight of a model 314 plane costs the company Rs 100, 000 and each flight of a model 535 plane costs Rs 150, 000. How many of each type of plane should be used to minimize the flight cost? Formulate this as a LPP.
Matching exercise
Match the items on the right to the items on the left.
Step - 1
Step - 2
Step - 3
Step - 4
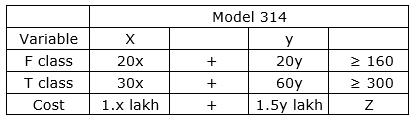
The above LPP can be presented in a table above.
The flight cost is to be minimized i.e; Min Z = x + 1.5y
the constraints
x ≥ 2 at least 2 planes of model 314 must be used
y ≥ 0 at least 1 plane of model .53.5 must be used
20x + 20y ≥ 160 require at least 160 F class seats
30x + 60y ≥ 300 require at least 300 T class seats
Solving the above inequalities as equations we get,
When x = 0, y = 8 and when y = 0, x = 8
When x = 0, y = 5 and when y = 0, x = 10
We get an unbounded region 8 - E - 10 as a feasible solution. Plotting the corner points and evaluating we have,

Since we obtained an unbounded region as the feasible solution a plot of Z (x + 1.5y< 9) is plotted.
Since there are no common points point E is the point that gives a minimum value.
Using 6 planes of model 314 & 2 of model 535 gives minimum cost of 9 lakh rupees.