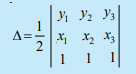Determinants

|A′| = |A|, where A′ = __________ of A
1. Inverse
2. transpose
3. determinate
4. None
If we interchange any two rows (or columns), then sign of determinant ______
1. changes
2. unchanged
3. Both a & b
4. None
If any two rows or any two columns are identical or proportional, then value of determinant is ________
1. Zero
2. one
3. two
4. ALL
If A = (aᵢᵣ)₃ₓ₃ then |K.A| =
1. k |A|
2. k² |A|
3. k³ |A|
4. None
Area of a triangle with vertices (x1, y1), (x2, y2) and (x3, y3) is given by
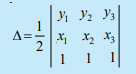
4. None
A (adj A) = (adj A) A = |A| I, where A is _____ matrix of order n.
1. singular matrix
2. square matrix
3. non-singular matrix
4. ALL
If AB = BA = I, where B is square matrix, then B is called ______ of A.
1. Square
2. inverse
3. transpose
4. adj
Evaluate
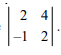
1. 7
2. 8
3. 9
4. 10
Evaluate
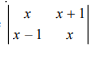
1. 0
2. 1
3. x
4. x²
Evaluate the determinant Δ
1. -52
2. -25
3. 52
4. 25
Evaluate
1. 1
2. 2
3. 3
4. 0
Find values of x for which
1. ± √2
2. ± 2√2
3. √2
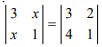
4. -√2
The value of the determinant remains _______ if its rows and columns are interchanged
1. changes
2. unchanged
3. None
4. negative
if A is a square matrix, then det (A) = det (A′), where A′ = ______ of A.
1. determinate
2. Inverse
3. transpose
4. None
1. 0
2. 1
3. 2
4. 3
Evaluate
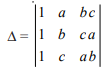
1. (a + b) (b – c) (c + a)
2. (a – b) (b – c) (c – a)
3. (a – b) (b + c) (c – a)
4. (a + b) (b + c) (c + a)
1. abc
2. 2abc
3. 3abc
4. 4abc
Find the area of the triangle whose vertices are (3, 8), (– 4, 2) and (5, 1).
1. 61/2
2. 61
3. 30
4. 20
A(adj A) = (adj A) A =
1. |A | I
2. A I
3. Both a & b
4. adjA
A square matrix A is said to be ______ if A = 0.
1. singular
2. non--singular
3. Null
4. none
A square matrix A is said to be ________ if A ≠ 0
1. singular
2. non-singular
3. Inverse
4. none
A square matrix A is ________ if and only if A is nonsingular matrix.
1. Adjacent
2. identity
3. invertible
4. None
A system of equations is said to be consistent if its solution ________.
1. exists
2. does not exists
3. undefined
4. none
A system of equations is said to be inconsistent if its solution ________.
1. exist
2. does not exist
3. undefined
4. none
A square matrix A has inverse if and only if A is ______
1. Singular
2. non-singular.
3. square matrix
4. none
For a square matrix A in matrix equation AX = B |A| ≠ 0, there exists _____
1. unique solution
2. no solution
3. may or may not be consistent
4. none
For a square matrix A in matrix equation AX = B |A| = 0 and (adj A) B ≠ 0, then there exists________
1. unique solution
2. no solution
3. may or may not be consistent
4. none
For a square matrix A in matrix equation AX = B |A| = 0 and (adj A) B = 0, then ______
1. unique solution
2. no solution
3. may or may not be consistent
4. none
Which of the following is correct
1. Determinant is a square matrix.
2. Determinant is a number associated to a matrix.
3. Determinant is a number associated to a square matrix
4. None of these
1. 0
2. 1
3. 2
4. 3

Determinants

Quiz