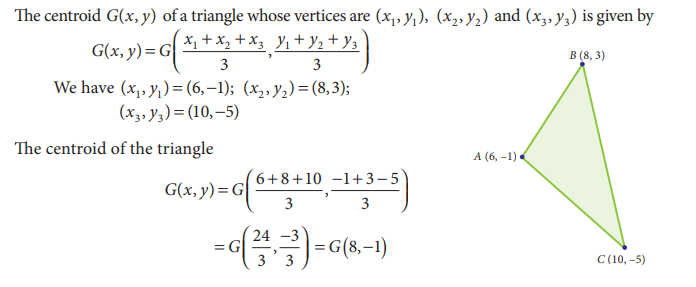COORDINATE GEOMETRY

Ø A coordinate geometry is a branch of geometry where the position of the points on the plane is defined with the help of an ordered pair of numbers also known as coordinates.

Quadrants:

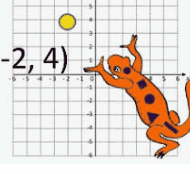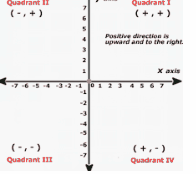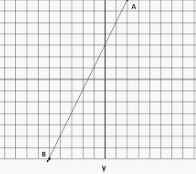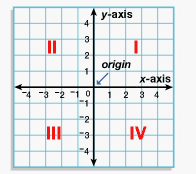
Ø The four regions into which a plane is divided by the axes of a Cartesian coordinate system.

Ø The quadrants are numbered counter clockwise one through four, beginning with the quadrant in which both the x- and y-coordinates are positive (usually the upper right quadrant).

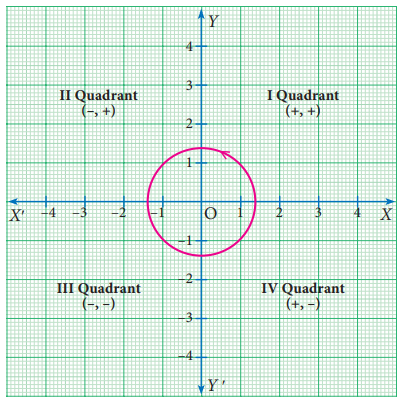
Ø Quadrant 1 – Points lies between 0 and 90

Ø Quadrant 2 – Points lies between 90 and 180

Ø Quadrant 3 – Points lies between 180 and 270

Ø Quadrant 4 – Points lies between 270 and 360

PROBLEMS

1. In which quadrant does the following points lie? (a) (3,–8) (b) (–1,–3) (c) (2, 5) (d) (–7, 3)

Solution:

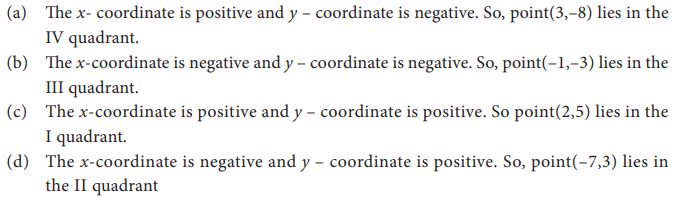
2. Find the distance between the points (–4, 3), (2,–3).

Solution:

3. Show that the points A(–4,–3), B(3,1), C(3,6), D(–4,2) taken in that order form the vertices of a parallelogram.

Solution:

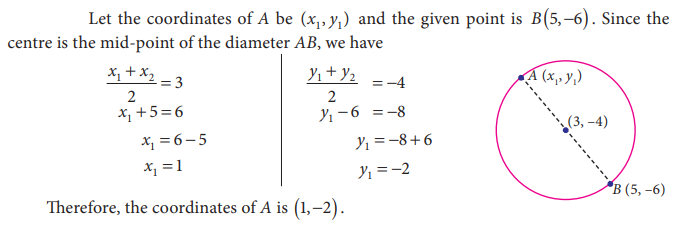
4. The point (3, -4) is the centre of a circle. If AB is a diameter of the circle and B is(5, -6) , find the coordinates of A.

Solution:

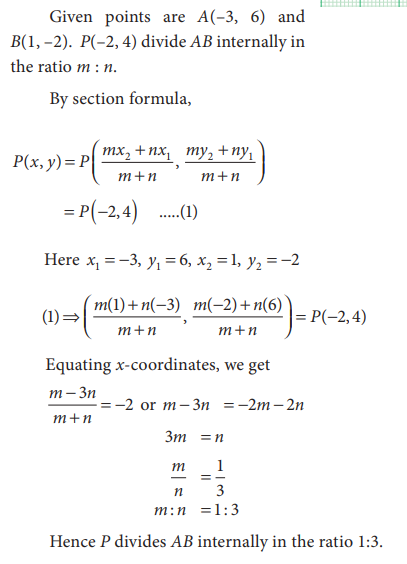
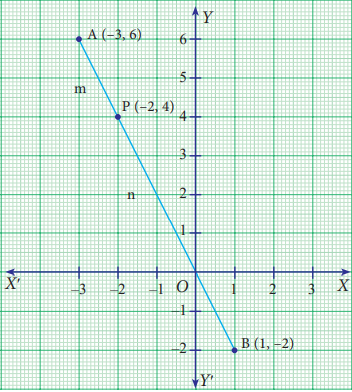
5. In what ratio does the point P(–2, 4) divide the line segment joining the points A(–3, 6) and B(1, –2) internally?

Solution:

6. Find the centroid of the triangle whose vertices are A(6, -1 ), B(8, 3) and C(10, −5).

Solution: