Electric Potential Due to a Dipole
An electric dipole is a pair of
two objects having equal and opposite charges, separated by a distance.
What is Electric Dipole?
Suppose
there are two charges of equal magnitude ‘q’, separated by a distance
‘d’. Let the first charge be negative and the second charge be positive.
This combination can be called as an electric dipole. Therefore, we
can say that an electric dipole is created by the combination of equal and
opposite charges by a separation of a certain distance.
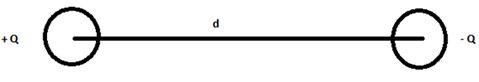
Now, the electric dipole moment
for this pair of equal and opposite charges is equal to the magnitude of the
charges multiplied by the distance between them.
Magnitude of
Electric Dipole Moment
![]() = q.
= q.![]()
Direction
of Electric Dipole Moment
Electric
dipole moment is a vector quantity; it has a defined direction which is from
the negative charge to the positive charge. Though, it is important to remember
that this convention of direction is only followed in Physics. In Chemistry,
the convention is taken to be opposite i.e. from positive to negative. The line
along the direction of electric dipole is called the axis of the dipole.
Electric
potential due to a Dipole (V)
Suppose
there are two charges –q, placed at A, and +q placed a B, separated by a
distance d, forming a dipole. Suppose the midpoint of AB is O.
The Electric potential due to a
dipole at any point P, such that OP = r will be:
V = ![]() p cos
p cos![]()
Case 1: If θ = 90°
Electric potential = V = 0
Case 2: If θ = 0°
Electric potential = V = ![]() p
p![]()
Potential energy of a dipole
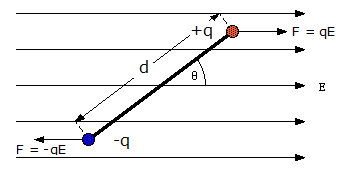
Consider
a dipole with charges q1 = +q and q2 = -q placed in a
uniform electric field as shown in the figure above. The charges are separated
by a distance d and the magnitude of electric field is E. The force
experienced by the charges is given as –qE and +qE, as can be seen in the figure.
As
we know that, when a dipole is placed in a uniform electric field, both the
charges as a whole do not experience any force, but it experiences a torque
equal to τ which can be given as,
![]()
This
torque rotates the dipole unless it is placed parallel or anti-parallel to the
field. If we apply an external and opposite torque, it neutralizes the effect
of this torque given by τext and
it rotates the dipole from the angle Ɵ0 to an angle Ɵ1 at
an infinitesimal angular speed without any angular acceleration.
The
amount of work done by the external torque can be given by
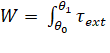 (
(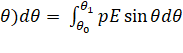
=
![]()
As
we know that the work done in bringing a system of charges from infinity to the
given configuration is defined as the potential energy of the system, hence the
potential energy U(Ɵ) can be associated to the inclination Ɵ of
the dipole using the above relation.
![]()
From
the above equation, we can see that the potential energy of dipole placed in an
external field is zero when the angle Ɵ is equal to 90° or when the dipole
makes an angle of 90°.
Considering
the initial angle to be the angle at which the potential energy is
zero, the potential energy of the system can be given as,
![]() =
= ![]()