The Second Law of Thermodynamics
After having understood the concepts of spontaneity
and entropy, now it is very easy to define second law of thermodynamics.
However, just as there are many ways of defining the first law of thermodynamics,
similarly there are many ways of defining the second law of thermodynamics.
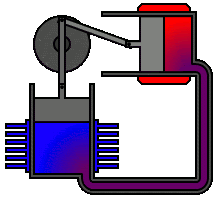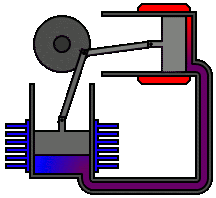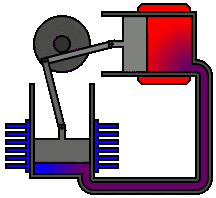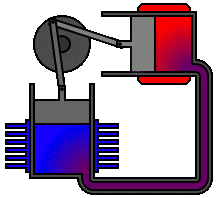
To
understand the most common statement of the second law, let us reconsider the
following spontaneous processes:
(i)
Cooling down of a cup of tea
(ii) Spreading of a drop of ink in water
(iii) Mixing of two gases
(iv) Flow of water
down a hill
We know
that once a cup of tea has cooled down to room temperature, it cannot become
hot by Similarly, the ink cannot separate out from water by itself or the gases
after mixing cannot separate spontaneously or water cannot go up-hill
spontaneously. In other words, the above processes cannot take place in the
reverse direction. Similarly, taking the example of a chemical reaction, we
know that reaction between NaOH and HCl solutions to form NaCl and H2O
is spontaneous but the reverse reaction i.e., reaction of NaCl
with H2O to give back the acid and the base does not take place on
its own. This gives first definition of the second law as follows:
All spontaneous processes
(or naturally occurring processes) are thermodynamically irreversible. (Ist def.)
The above processes can, however,
be brought about in the reverse direction by using some external agency, e.g.,
water can be made to go uphill with an electric motor, tea can be made hot again
by heating. Hence, the second law can be defined in an alternate manner as
follows:
Without the help of an
external agency, a spontaneous process cannot be reversed, e.g., heat cannot by
itself flow from a colder to hotter body. (2nd def.)
Further,
the first law states that when heat is converted into work, the work obtained
is equivalent to the heat absorbed. However, it has been seen from experience
that the heat absorbed cannot be completely converted into work without leaving
some change in the system or the surroundings. For example, the heat produced
in a locomotive is not fully utilized in running the train. A part of it is
lost to the surroundings or wasted in overcoming the friction. Hence, the
second law is also stated as follows:
The complete conversion
of heat into work is impossible without leaving some effect elsewhere (3rd
def.)
In terms of entropy (as
already discussed) the definition of the second law may be given as follows:
All spontaneous processes
are accompanied by a net increase of entropy, i.e., for all the spontaneous
processes, the total entropy change (sum of the entropy changes of the system
and the surroundings) is positive (4th def.)
Further,
since all naturally occurring processes are spontaneous and are accompanied by
a net increase of entropy, hence the second law is also sometimes defined as
follows:-
The entropy of the
universe is continuously increasing (5th def.) Thus, the main ideas of the
first and the second law of thermodynamics may be summed up in following
statement:
“The energy of the
universe is constant whereas the entropy of the universe is continuously
increases and tends to a maximum”.
The second law of thermodynamics states that for
any spontaneous process, the overall ΔS must be greater than or equal to
zero; yet, spontaneous chemical reactions can result in a negative change in
entropy. This does not contradict the second law, however, since such a
reaction must have a sufficiently large negative change in enthalpy (heat
energy). The increase in temperature of the reaction surroundings results in a
sufficiently large increase in entropy, such that the overall change in entropy
is positive. That is, the ΔS of the surroundings increase enough because
of the exothermicity of the reaction so that it
overcompensates for the negative ΔS of the system.
Since the overall ΔS = ΔSsurroundings +
ΔSsystem, the overall change in
entropy is still positive.
Problems
1. When disorder of a
system increases, the change is said to be
A)
Exothermic
B)
Non-spontaneous
C) Endothermic
D)
Spontaneous
Solution:
When ΔS=+ve the change is spontaneous
2.
The spontaneous flow of heat is always
A)
From low to high pressure
B)
From high to high pressure
C)
Unidirectional from lower temperature to higher temperature
D)
Unidirectional from the higher to lower temperature
Solution:
Heat is
always flow from the higher to lower temperature.
3. Mixing of
non-reacting gases is generally accompanied by
A)
Decrease in entropy
B)
Increase in entropy
C)
Change in enthalpy
D) Change
in free energy
Solution:
Mixing of
non-reacting gases increases randomness and so increase entropy.