Raoult’s Law
Statement:
The partial
vapour pressure of any volatile component of a solution is the product of
vapour pressure of that pure component and the mole fraction of the component
in the solution.
Explanation:
Let us
consider a solution containing two volatile components say A1 and A2,
with mole fractions 
and 
respectively. Let 
and 
be the
vapour pressures of the pure components A1 and A2
respectively, then by Raoult’s law
=
and 
=
The total
vapour pressure of the solutions of two volatile components is the sum of
partial vapour pressures of the two components.
= +
= +
But + = 1
Thus = 1 –
Hence, = + (1 – )
∴ = + – 
∴ = + ( – )
The solution which obeys Raoult’s law over the entire range of concentration is
called an ideal solution. If a solution does not obey Raoul’s law are called non-ideal
solutions.
Raoult’s Law for a Solution of Non-Volatile Solute:
Let us
consider a solution containing two volatile component A1 and
non-volatile component A2, with mole fractions and respectively.
Let and be the vapour pressures of the pure components
A1 and A2 respectively. Now component A2 is
non-volatile, hence it will not contribute to vapour pressure. Thus = 0. We
have
= + ( – )
p = 0 + ( – 0)
p = 
Thus vapour pressure of a
solution of non-volatile solute is the product of vapour pressure of
pure solvent and mole fraction of the solvent, which is Raoult’s law.
The equation shows that vapour
pressure of the solution p < ,
i.e., there is lowering of the vapour pressure of the solution.
The lowering of vapour pressure is given by
Δ
= –
∴ Δ = –
∴ Δ = (1 – )
But + = 1
Thus = 1 –
∴ Δ = – 
In a solution containing several
non-volatile solutes, the lowering of the vapour pressure depends on the sum of
the mole fraction of different solutes.
Now, the
relative lowering of vapour pressure is given by
= 
= 
=
This relation proves that the
lowering of vapour pressure is colligative property because it depends on the
concentration of non-volatile solute.
Raoult’s Law as Special Case of Henry’s Law:
By Raoult’s law, we have
p = 
------ (1)
By Henry’s
law, we have
p = 
------ (2)
If we compare the two equations
for Raoult’s law and Henry’s law, it can be seen that
the partial pressure of the volatile component or gas is directly proportional
to its mole fraction in solution. Only the proportionality constant 
differs from p10 . Thus,
Raoult’s law becomes a special case of Henry’s law in which KH is
equal to .
Relation Between Molar Mass of Solute and Lowering of Vapour
Pressure:
Let W2 g of the solute
of molar mass M2 be dissolved in W1 g of the solvent of
molar mass M1. The number of moles of solvent and solute are given
by
= 
and 
= 
respectively
The mole
fraction of solute is given by
= 
= 
∴ =
=
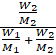
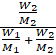
Now for
dilute solutions n2
<< n1. Hence n2 can be neglected.
∴ =
= 
= 
= × 
∴ =
= 
This is the relation between the
molar mass of solute and lowering of vapour pressure.
|
|
|
|
